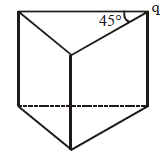
Solution:

To calculate the electric flux passing through the prism:

1. Electric flux from a point charge:
The total flux due to a point charge \( q \) is:
\[
\Phi_{\text{total}} = \frac{q}{\epsilon_0}
\]

2. Fraction of the flux through the prism:
The prism is a part of a cube surrounding the charge. Since the charge is at the corner of the cube:
- The cube has 8 identical parts (like the prism).
- The flux through the prism is \( \frac{1}{8} \) of the flux through the cube.

3. Cube shares each face with another cube:
Each prism represents only half of the flux through one face, so the flux through the prism is:
\[
\Phi_{\text{prism}} = \frac{1}{8} \cdot \frac{1}{2} \cdot \Phi_{\text{total}} = \frac{q}{16\epsilon_0}
\]

Thus, the flux through the prism is:
\[
{\frac{q}{16\epsilon_0}}
\]